What is the failure domain in a reliability analysis?

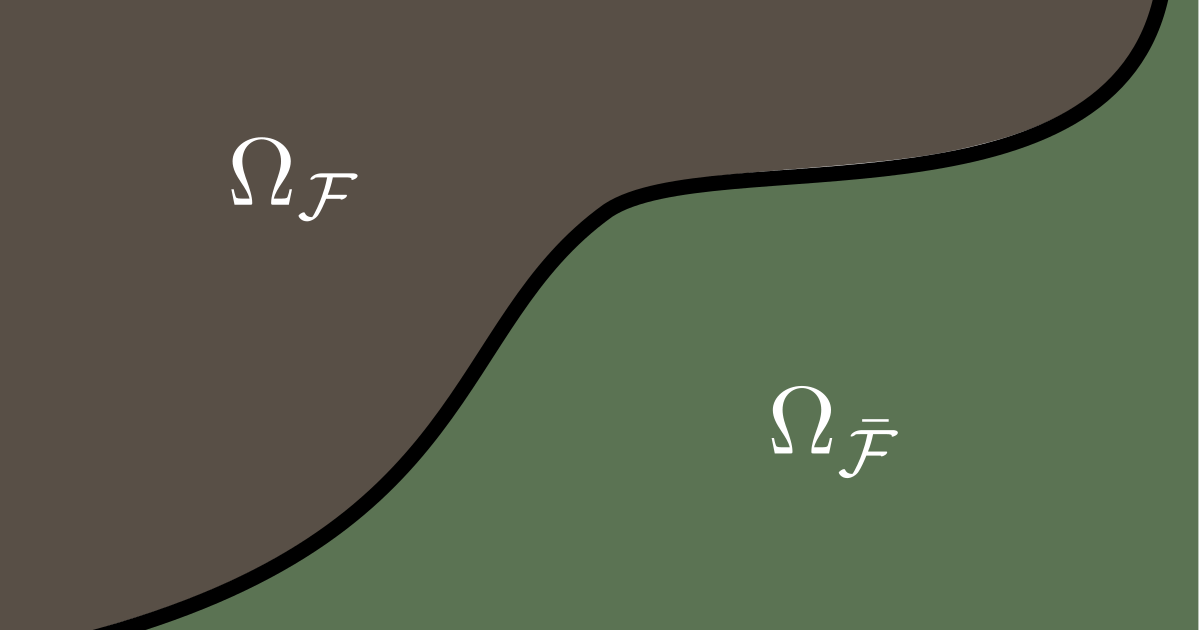
In structural reliability analysis, the failure domain $\Omega_\mathcal{F}$ consists of all samples $\mathbf{x}$ for which the limit-state function $g(\mathbf{x})$ is smaller or equal than zero; i.e., $\Omega_\mathcal{F} = \{\mathbf{x}\in\Omega|g(\mathbf{x})\le 0\}$, with sample space $\Omega \subseteq \mathbb{R}^M$. The probability of failure is, thus, defined as the integral of the joint probability density function $f_\mathbf{X}(\mathbf{x})$ of $\mathbf{X}$ over the entire failure domain $\Omega_\mathcal{F}$: $$
p_f = \Pr(\mathcal{F}) = \int_{\Omega_\mathcal{F}} f_\mathbf{X}(\mathbf{x}) \, \operatorname{d}\mathbf{x}\,.
$$

By applying the transformation $T:\mathbf{X}\to\mathbf{U}$ to all points in $\Omega_\mathcal{F}$, the failure domain $\mathbf{U}_\mathcal{F}=\left\{\mathbf{u}\in\mathrm{R}^N|G(\mathbf{u})\le0\right\}$ in standard Normal space is obtained.

A numerical challenge in structural reliability analysis is that the shape of the failure domain is typically not known explicitly. Thus, for some sample $\mathbf{x}$, we usually cannot say whether $\mathbf{x}$ belongs to the failure domain $\Omega_\mathcal{F}$ or not, without evaluating the limit-state function $g(\mathbf{x})$ for $\mathbf{x}$.